Friction-induced instability

Figure 1. Rod-on-plate setup

Figure 2. Simulation of the rod-on-plate setup

Figure 3. Friction force with respect to displacement. Parametric uncertainty-based simulation (blue), measurement (red)

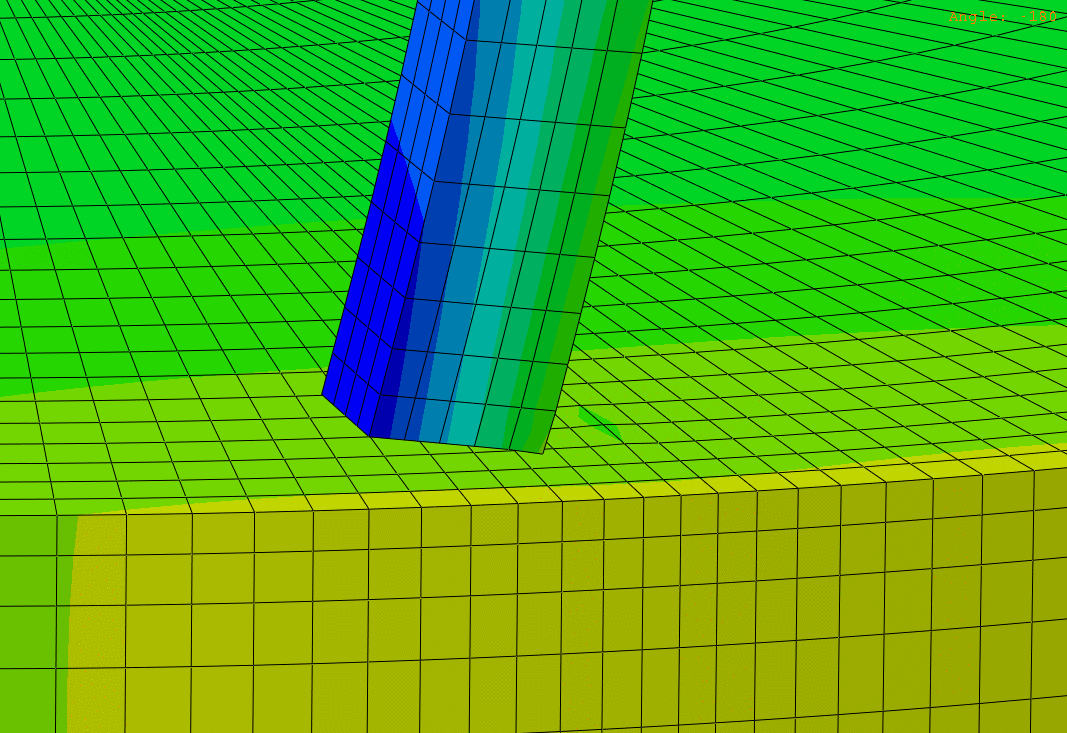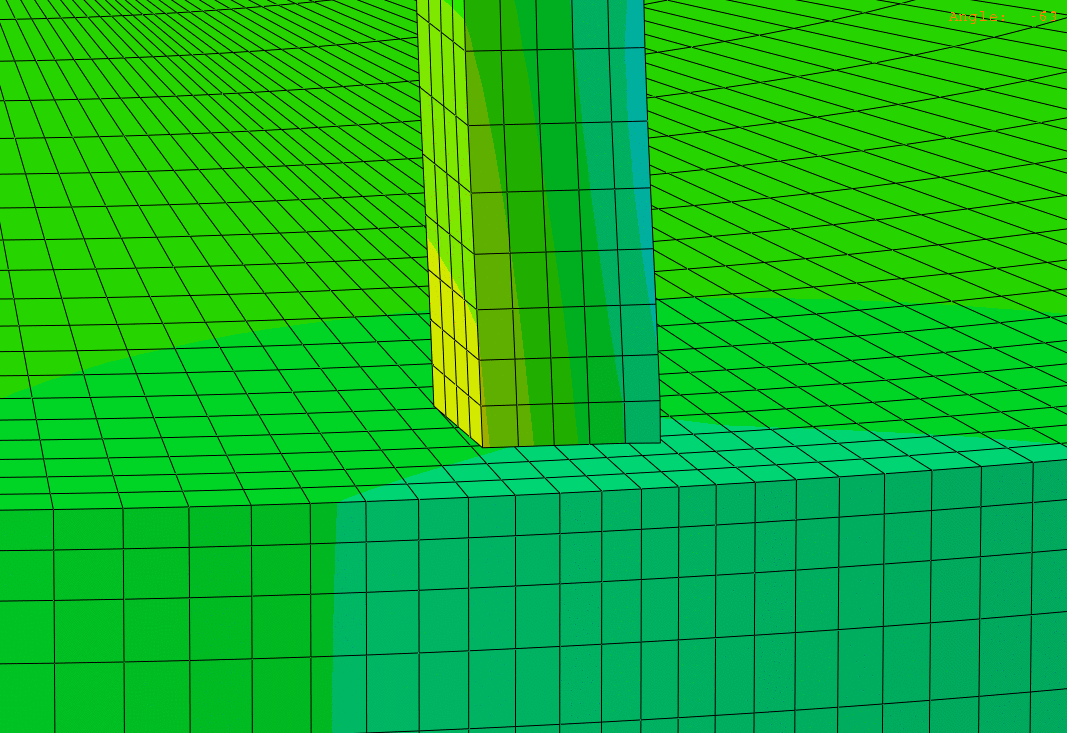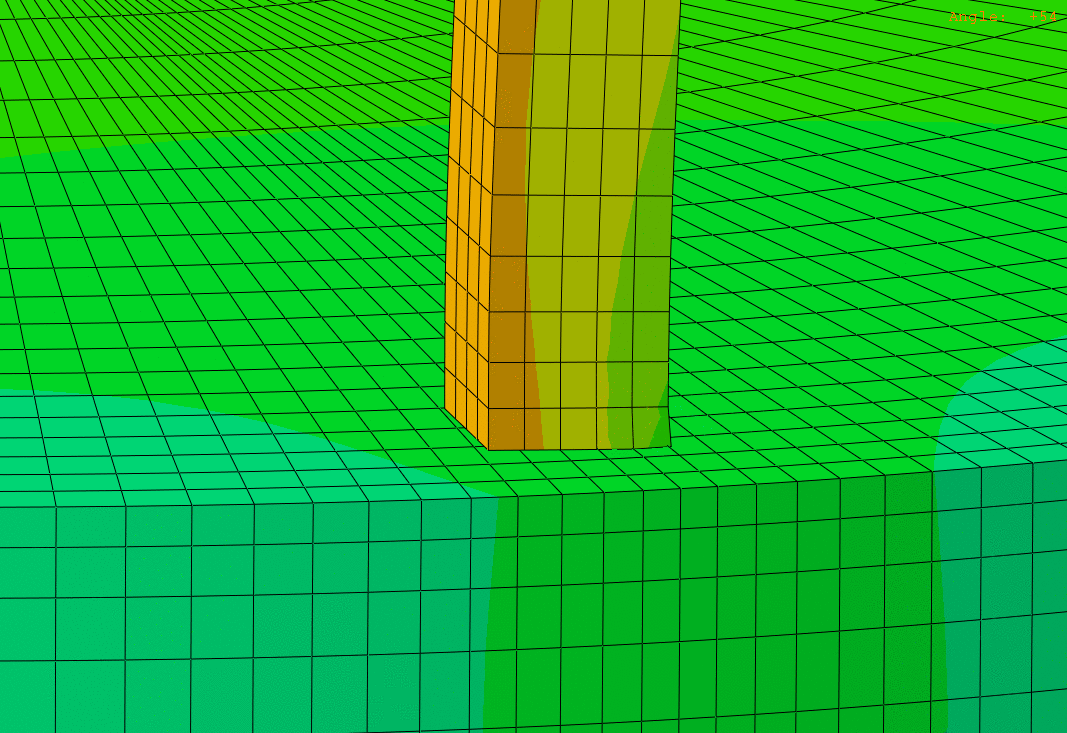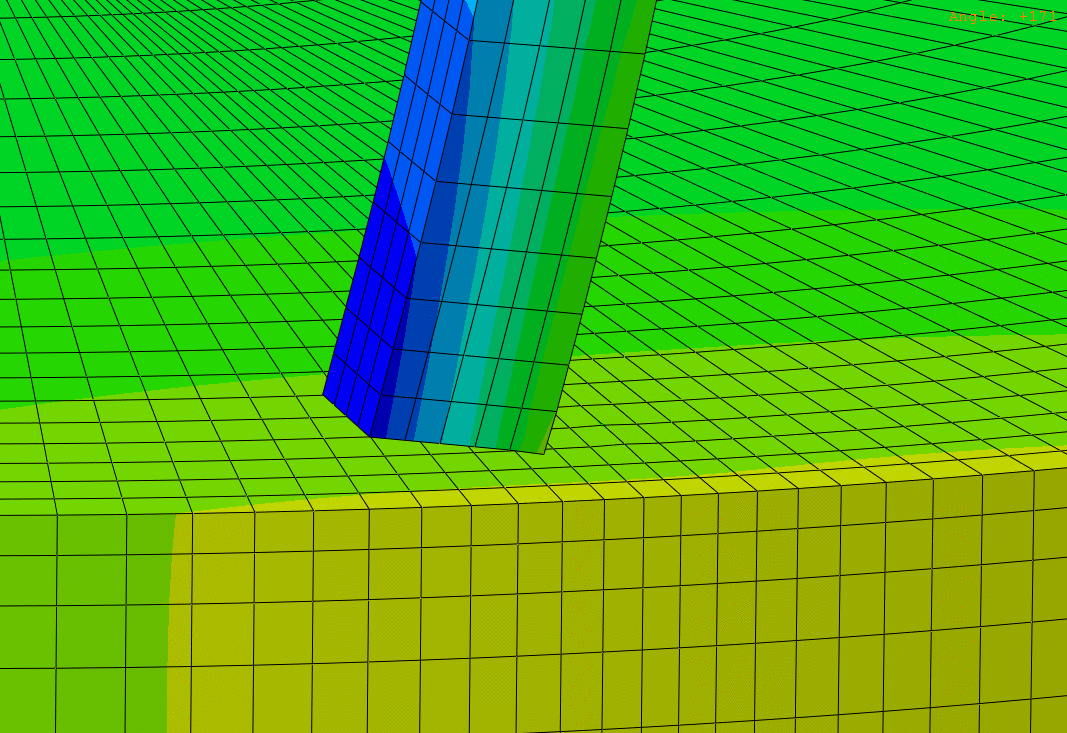
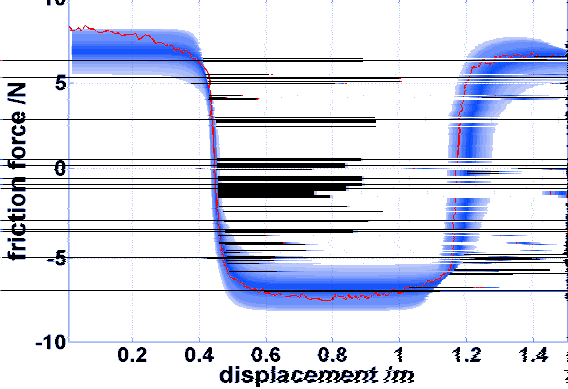
In the field of machine design, sliding contacts of components are frequently occurring, as for examples in door hinges, guide slide bearings, or clutch and brake systems. If certain preconditions are satisfied, the friction between the parts initiates an excitation process, which may result in an oscillating motion of the structure. This behavior is caused by an instability, which might affect the functionality of the system or lead to an inconvenient acoustic disturbance.

Experimental analysis

The phenomenon of friction-induced excitation can be examined using a rod-on-plate setup (Figure 1.). Hereby a load is applied to the rod, pushing it in against the plate. The plate is rotating about its origin, causing a sliding motion of the pin. In a certain configuration of the two components, the instability emerges, leading to the oscillating motion of the system. To analyse this phenomenon, the oscillation behavior of the system is investigated in two stages: when the plate is at rest, and when it is in motion. For the experimental analysis, different measurement techniques are applied: Laser-Doppler-Vibrometer, piezoelectric acceleration sensors, impact hammer and microphones for measuring the noise pressure level.

Numerical simulation

The friction-induced instability of the rod-on-plate setup can also be analysed using modeling techniques, such as the finite element method or multibody system modelling (Figure 2.). To ensure the authenticity of the simulation, the models of the system are updated using measurements, which result from the experiments. The subsequent numerical analysis enables a detailed investigation of the phenomena, which, when done experimentally, involves a tremendous efforts, or may not be possible at all.

Inclusion of parametric uncertainties

Numerical models of physical system are always subject to uncertainties. These can arise due to lack of knowledge, simplifications or idealization. The resulting deviation from the physical system manifests in parametric uncertainties. In order to analyse the influence of parametric uncertainties on the instability of the rod-on-plate system, an advanced fuzzy arithmetical technique is deployed. Hereby the parametric uncertainties are represented by fuzzy numbers. After the evaluation of a given problem, the results are then also of fuzzy-valued type, as displayed in Figure 3.

Related Pages

• Uncertainties in Dynamical Systems

Contacts

• apl. Prof. Dr.-Ing. Michael Hanss
• M.Sc. Andreas Hanselowski